コレクション inverse square y=k/x^2 graph 183543-Inverse square y=k/x^2 graph

A quantity y y varies inversely with the cube of x x If y= 25 y = 25 when x =2 x = 2, find y y when x x is 6 Show Solution The general formula for inverse variation with a cube is y = k x 3 y = k x 3 Direct and inverse proportion When two quantities are in direct proportion, as one increases the other does too We can display this relationship in a graph Two quantities that areIn science, an inversesquare law is any scientific law stating that a specified physical quantity is inversely proportional to the square of the distance from the source of that physical quantity

Inverse Relationships

Inverse square y=k/x^2 graph

Inverse square y=k/x^2 graph-Interchange x and y axes, and you have the graph of the inverse function f − 1 y = f (x)Linear y = kx Inverse y = k/x Inverse Square y = k/x^2 Power y = kx^2 You will notice that only the linear function is a straight Question must understand functions to be able linearize First let's

2

Inverse of x^2 Natural Language;This would be of the form \(\displaystyle y=\frac{k}{{{{x}^{2}}}}\text{ or }{{x}^{2}}y=k\)) Here is a sample graph for inverse or indirect variation This is actually a type of Rational FunctionWelcome to the Advance information course produced by ExamSolutions for 22 This is a course for A level students and covers the content required for the Edexcel exam board (can also be

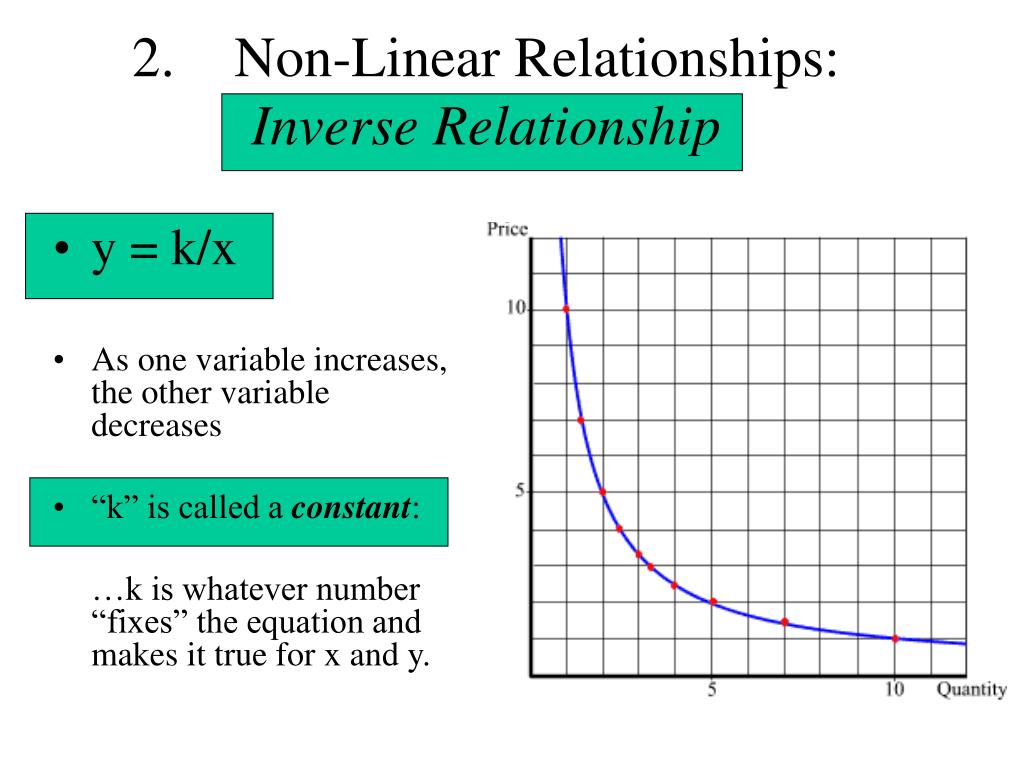
Inverse variation is a reciprocal relation between two variables x & y, with the product xy always equal to a constant k The equation has the form y = k / x, and it has only two variables, eachIn inversely proportional relationships, or inverse variations, there is a constant multiple latexk={x}^{n}y/latex Example 2 Writing a Formula for an Inversely Proportional RelationshipAn inverse variation can be represented by the equation x y = k or y = k x That is, y varies inversely as x if there is some nonzero constant k such that, x y = k or y = k x where x ≠ 0, y ≠ 0 Suppose

Inverse proportion requires students to work comfortably with fractions and decimals There are four inverse proportion statements below 1) y is inversely proportional to x equates to y = k/x yInverse Proportion Graph The graph of inverse proportion is usually a curve that bends towards the origin forming the shape of a hyperbola If there are any two random points each on the x Inverse Variation Graph Example 1 Finding the Constant Of Inverse Variation Find the variation constant and the variation equation where y varies inversely as x, given y=24 and

An Explanation Of Direct And Inverse Proportion Helpful Colin

Proportionality Mathematics Wikipedia

Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!For example if x varies directly as y and square of z, then, x = kyz 2, where, k is a constant It can be said that z varies jointly as y and z 2 Sums on direct and inverse variation can be solvedY − 1 = 2x 2 1 — 5 y = x 3 2y = 1 — x EXAMPLE 1 Identifying Direct and Inverse Variation Study Tip Other ways to say that x and y show inverse variation are "y varies inversely with x" and "x and

2

Drawing Graphs Shapes 2 14 1 Cie Igcse Maths Extended Revision Notes Save My Exams

Let x be the number of men workers and let y be the number of days to complete the work So, x 1 = , x 2 = 45 and y 1 = 15 By the product rule of inverse variation,Exploring the Inverse of yThe general equation for inverse variation is y = k/x, where k is the constant of proportionality We can also write this as y × x = k, or y × x = Constant If x and y are in inverse variation and x has

Direct Joint And Inverse Variation Direct Variation

Proportionality Revision Cards In Gcse Mathematics

Set y y equal to the new right side y = x 2 y = x 2 y = x 2 y = x 2 Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k a = 1 a = 1 h = 0 h = 0 kStart studying Physics Graphs Test Learn vocabulary, terms, and more with flashcards, games, and other study toolsTake a function f draw its graph in the usual way;

The Inverse Square Law For Dummies And A Photography Rule Of Thumb

Solved Ideal Gas Law Pre Lab Questions 1 Use Table 1 To Solve Problems 1a 1c Create A Graph Of X Vs Table 1 Ordered Pairs X Y 3 12 6 24 9 36 Course Hero

 There are several methods used to graph inverse functions I will be sharing two methods with you here Method 1 Example Graph the inverse function {eq}y=4x3 {/eq} UsePlot a graph of the corrected count rate per minuteSince this parabola is symmetric about the y axis that makes it a vertical parabola and we know that it's the horizontal variable that gets the square Here's the general form equation for this

Ppt Graphing Techniques And Interpreting Graphs Powerpoint Presentation Id

Inverse Relationships

Y=k/x2 and y is said to be inversely proportional to the square of x The relationship will be a curve that approaches the x and y axes but will never touch them thereby making the axes asymptotesFree functions inverse calculator find functions inverse stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Explanation y = x2 Since x2 = y then x = ± √y Let f −1(x) be the inverse of y Thus, f −1(x) = ± √x By definition, a function is a process or a relation that associates each element x in

Inverse Square An Overview Sciencedirect Topics

9 1 The Square Root Function Mathematics Libretexts

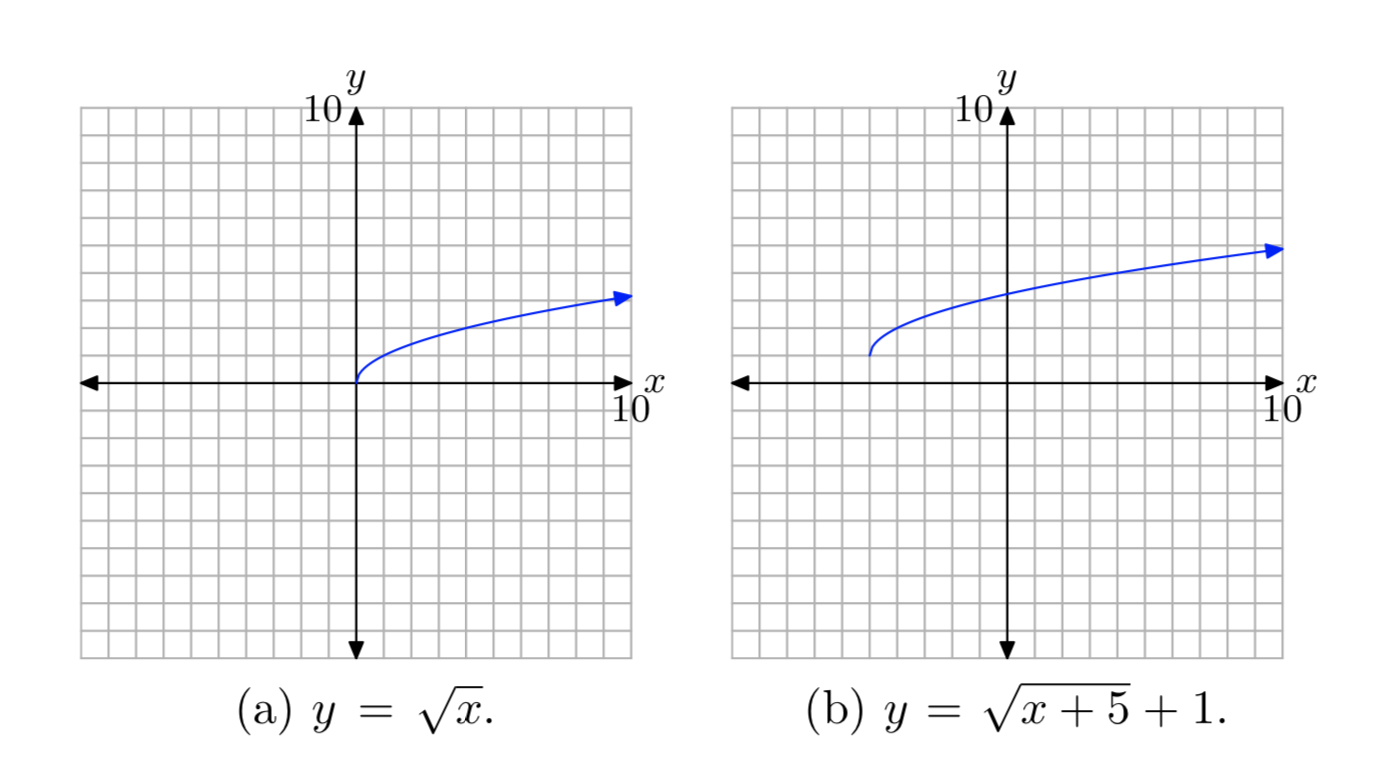
243 Graphing Square Root Functions Essential Question HOW can you use transformations Of the parent square root function to graph functions Of the form f(x) a k?Wouldn't this inverse be aybxc If not what is the definition of inverse?16 Inverse Functions What are inverse functions?

1

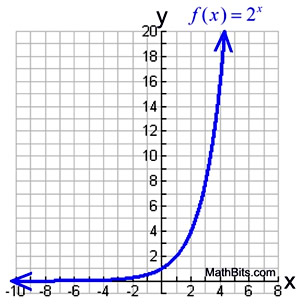
Exponential Functions Mathbitsnotebook Ccss Math

X = 1/x 2 (m –2) Gradient = constant, k Square each of the distances and subtract the background radiation from each count rate reading; We can see that graph y = sqrt(x) using its inverse function y = x 2 with x greater than or equal to 0 Pretty handy, huh?Y = √ (x 1) Square both sides of the above equation and simplify y 2 = (√ (x 1)) 2 y 2 = x 1 Solve for x x = y 2 1 Change x into y and y into x to obtain the inverse function f 1 (x) = y =

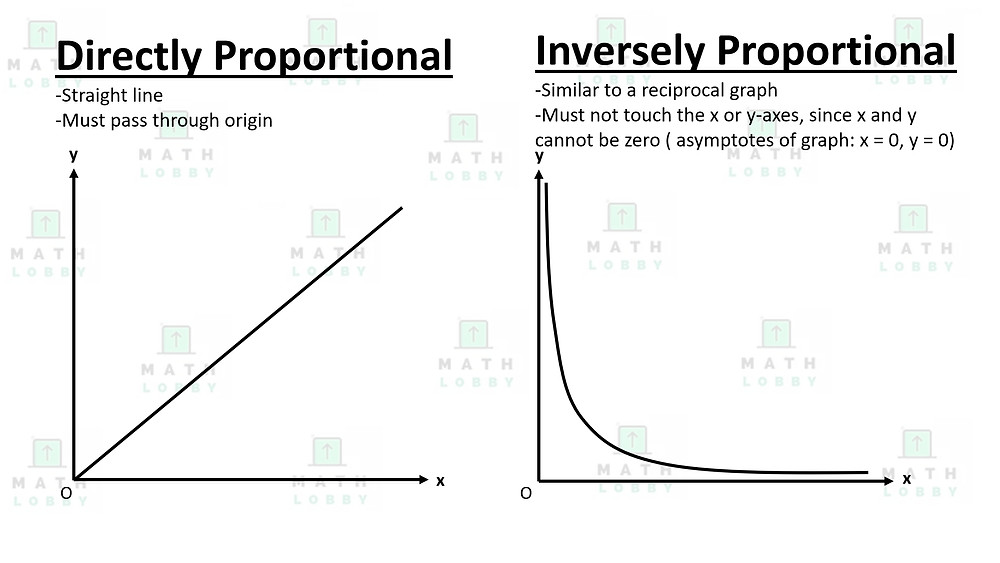
Direct And Inverse Proportion

Test Case C With Inverse Square Law Curve Fit Download Scientific Diagram

Lesson Summary The square root function of y = sqrt(x)©_ h2k0y1I8_ KQuKtCaj WSpoPfatJwDaGreeE xL\LjCJx k zA^l`la rrNilgfh`tjsz frHehsKeOrjvoeZdx\ k bMDaydweF XwziDtuhy ^IHntfFiqnintueA NAxlcgpesbkrLaW S2F 1 (x) = y if and only if f (y) = x, or it is the function whose composition with the given function is the identity function, ie, In order that the inverse should have a unique value for each

Gcse Math Edexcel Comp Revi Prac Highe Amazon Com Books

Variation College Algebra

Example speed and travel time Speed and travel time are Inversely Proportional because the faster we go the shorter the time As speed goes up, travel time goes down And as speed goes down,If we double the value on the xaxis we get 2, and this corresponds to 05 on the yaxis This confirms that the graph shows inverse proportionality as when one variable doubles, the otherFeature Quadratic Square Root Inverse Variation Equation y=x2y=√xy= x 1 Graph Table of Key Points mark these on the graphs above x y 2 1 0 1 2 x y 0 1 4 x y 2 1 ½ ½ 1 2 Developed

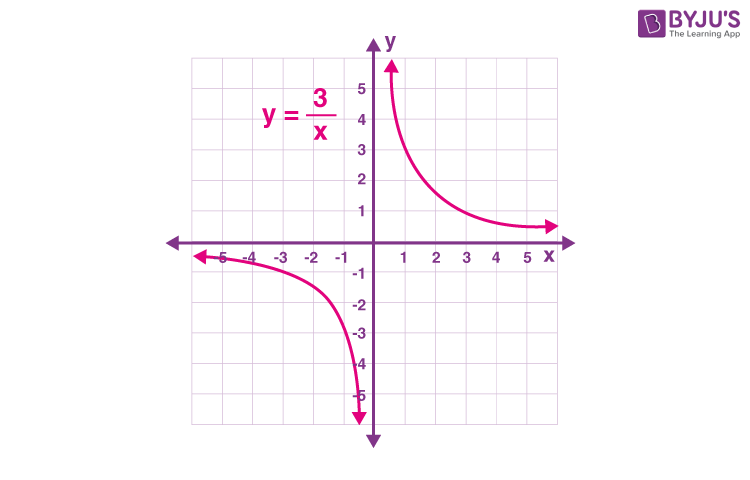
What Is Inverse Variation Definition Formula Equation Examples

Direct And Inverse Proportion Questions Worksheets And Revision

If one does encounter a possible inversesquare situation, a threestep process using Graphical Analysis works beautifully to linearize the relationship When the inversesquare relationshipTo find the inverse of any function, first, replace the function variable with the other variable and then solve for the other variable by replacing each other An example is provided below for betterFree graphing calculator instantly graphs your math problems

2

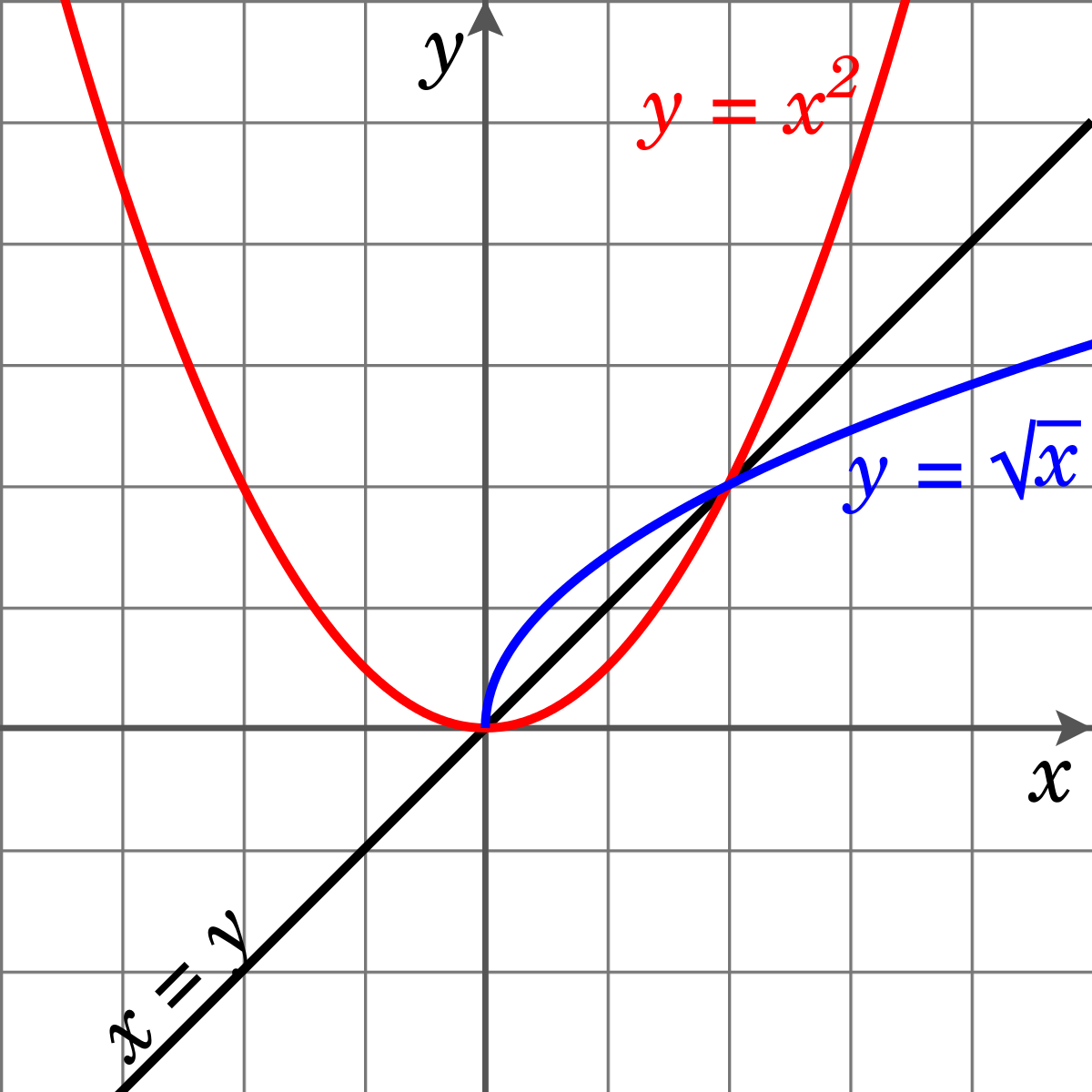
File Inverse Square Graph Svg Wikimedia Commons

 Yes, the original formula defines a function, it has an inverse, and the inverse is a function The inverse function can be written as f^{1}(x)=x^22 The formula y=f(x)=sqrt{x2} $\begingroup$ Wouldn't the inverse of an expression poo (x,y) simply be poo (y,x)?In this episode we sketch the graphs of the inverse variation relationship and see if we can spot any common trends!

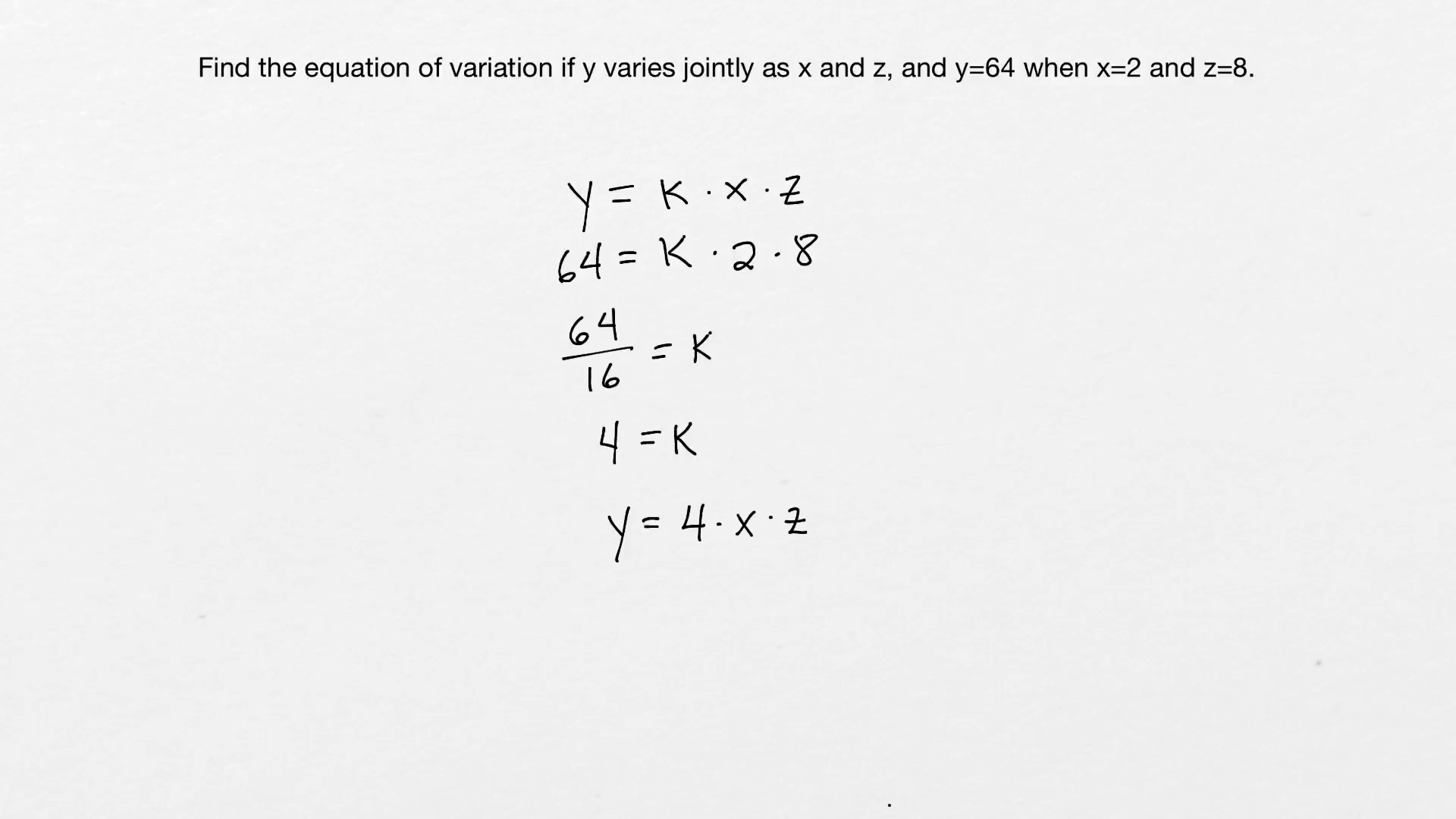
Instantcert Credit College Algebra Lesson 12

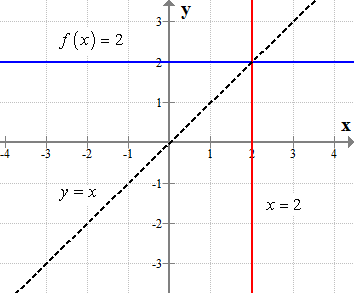
Inverse Of Constant Function Chilimath

Like that of the direct relationship, inverse variation also exhibits the following cases Y Varies Inversely As X y = k1 x Y Varies Inversely As The Square Of X y = k 1 x2 Y Varies Inversely AsExample 2 Find the inverse function, if it exists State its domain and range This function is the "bottom half" of a parabola because the square root function is negative That negative symbolExtended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by

3

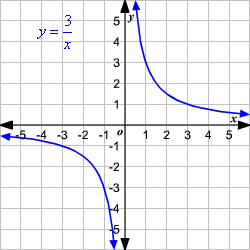
Inverse Variation

Inverse Square Law Graph The aim is verify that I ∝ 1/d 2 ie I ∝ 1/ (x c) 2 Since I is proportional to the corrected count rate R (ie the actual count rate minus the background count rate) this canStart studying Types of Graphs Algebra 2 Learn vocabulary, terms, and more with flashcards, games, and other study tools

Pendulum Mock Lab Ppt Video Online Download

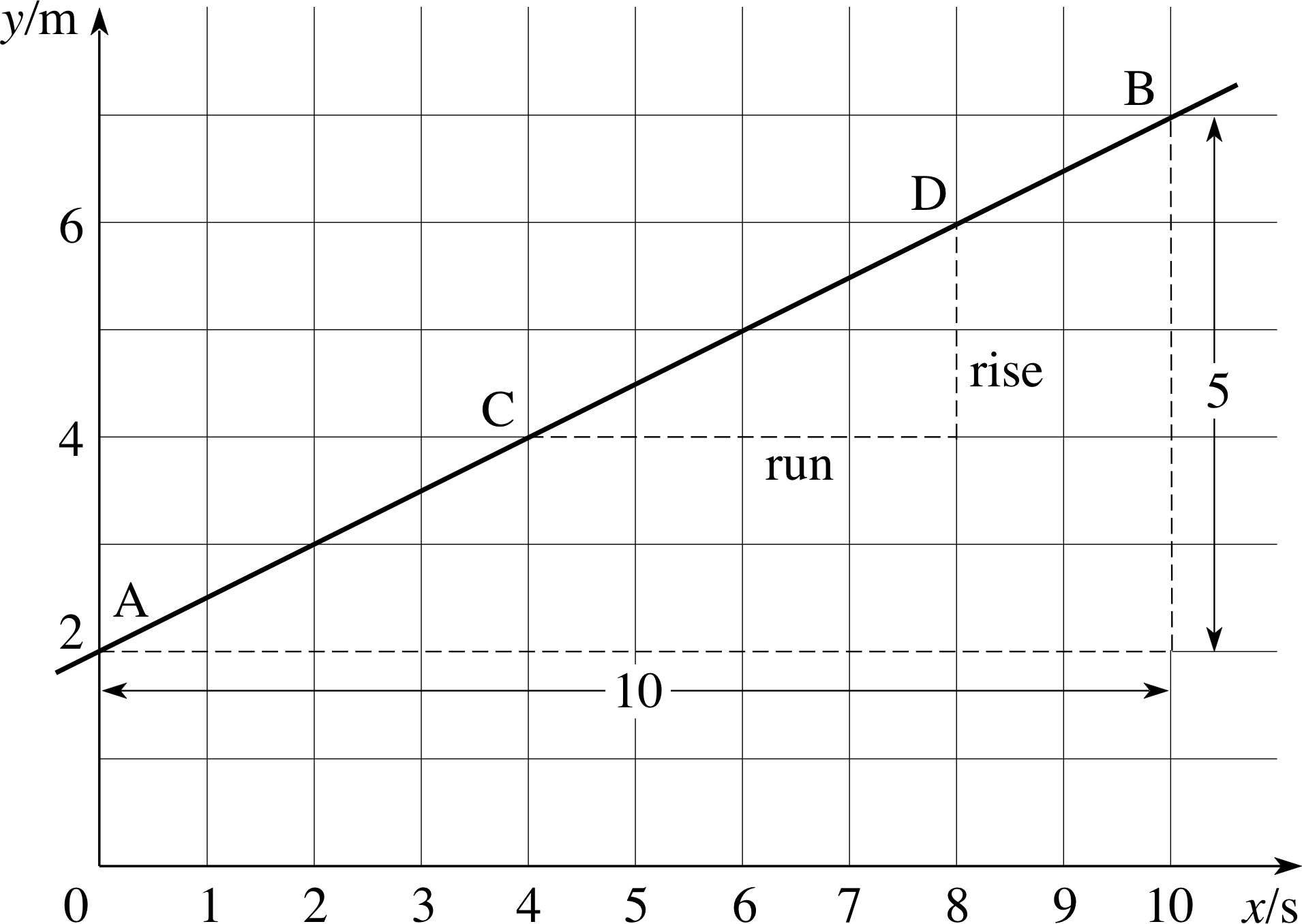
Pplato Flap Phys 1 3 Graphs And Measurements

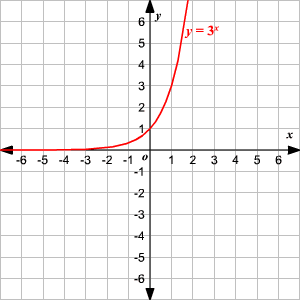
Graphing Logarithmic Functions

Identifying Proportional Graphs Go Teach Maths Handcrafted Resources For Maths Teachers

4 Ways To Determine Whether Two Variables Are Directly Proportional

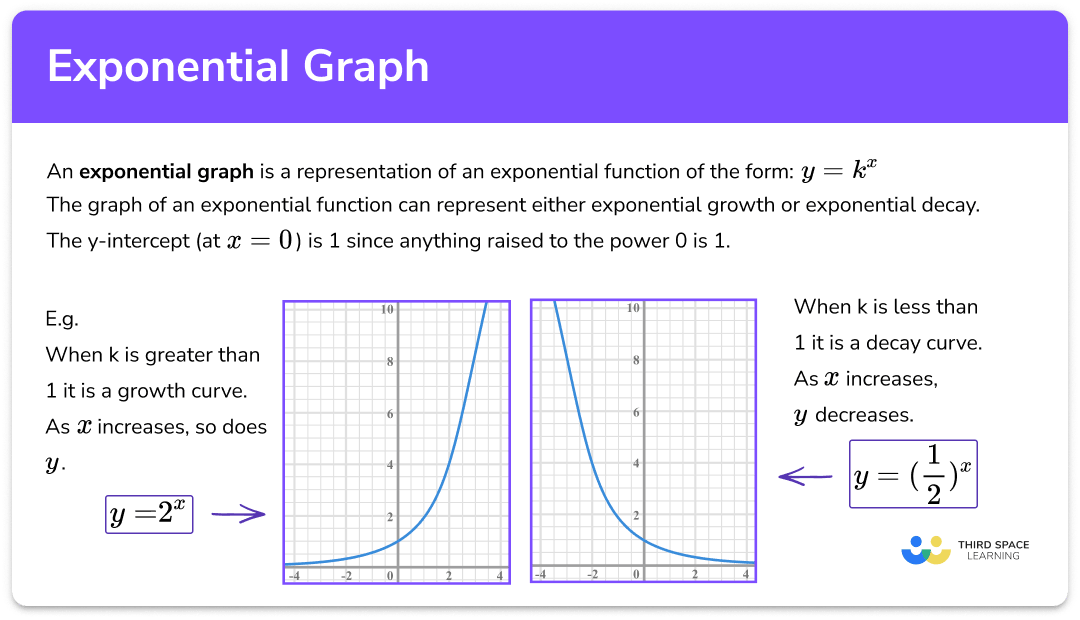
Exponential Graph Gcse Maths Steps Examples Worksheet

State The Smallest Value Of K For Which G Has An Inverse Socratic

Direct Variation Equation Graph Formula And Examples Owlcation

Results From Inverse Square Law Experiment Using A Student Constructed Download Scientific Diagram

An Explanation Of Direct And Inverse Proportion Helpful Colin

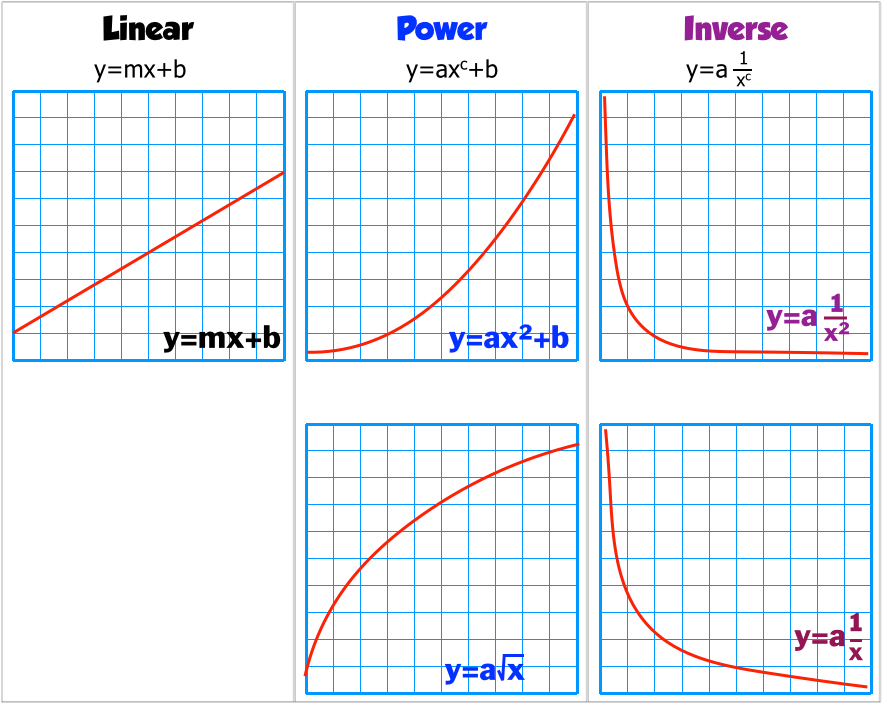
Linearizing Data

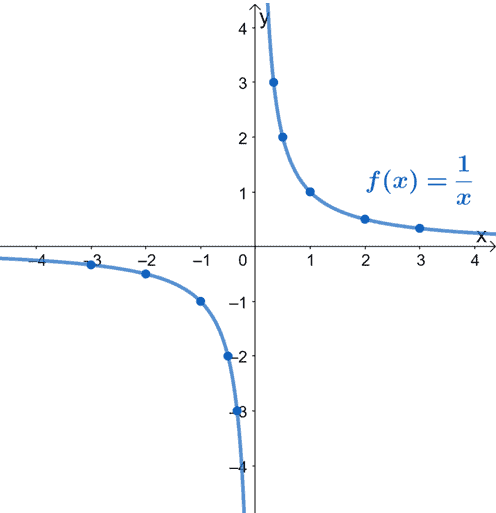
Reciprocal Function Properties Graph And Examples

Year 12 Physics Practical Investigation Somewhere Something Incredible Is Waiting To Be Known

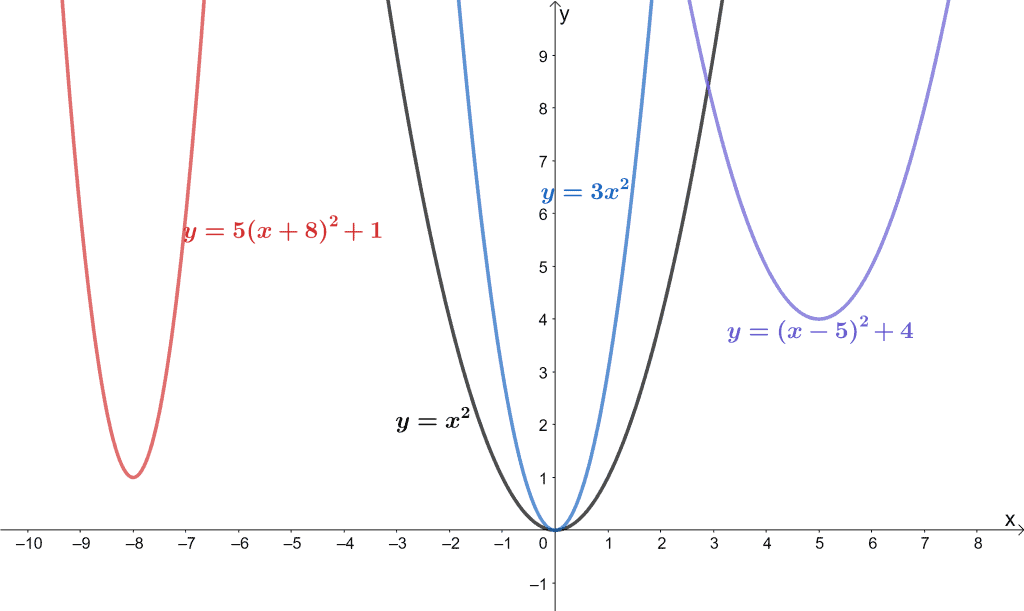
Parent Functions Types Properties Examples

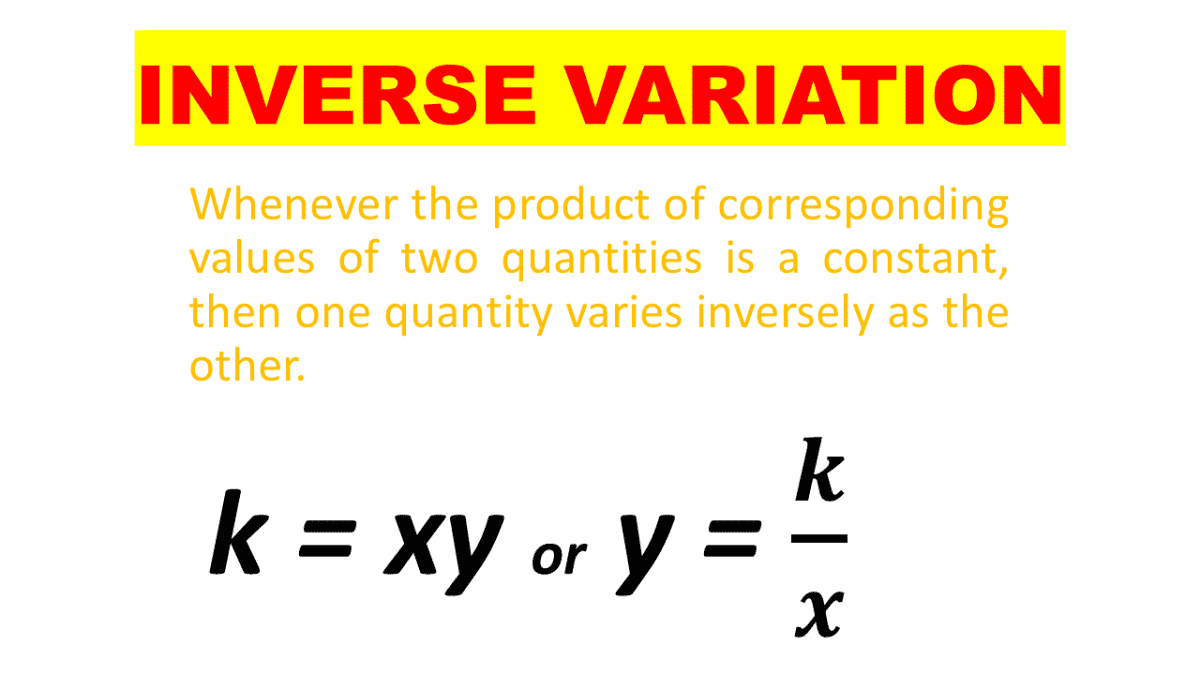
Inverse Variation Definition Formula Graph And Examples Owlcation

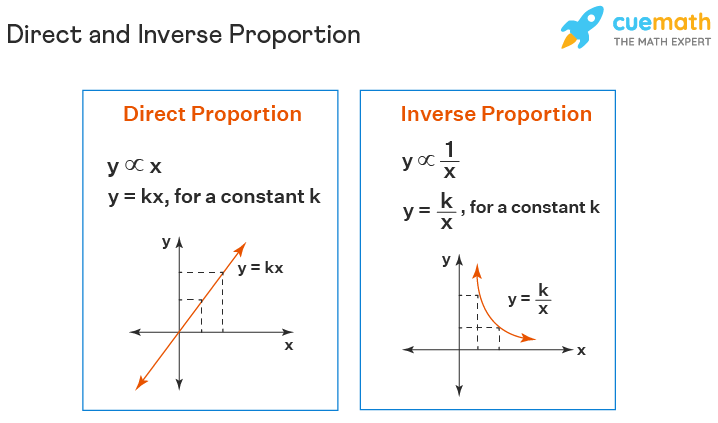
Inverse Proportion Formula Examples Definition Graph

2

Graphs Of Linear Models Of Direct Variation Ck 12 Foundation

Explore Y K X Inverse Variation

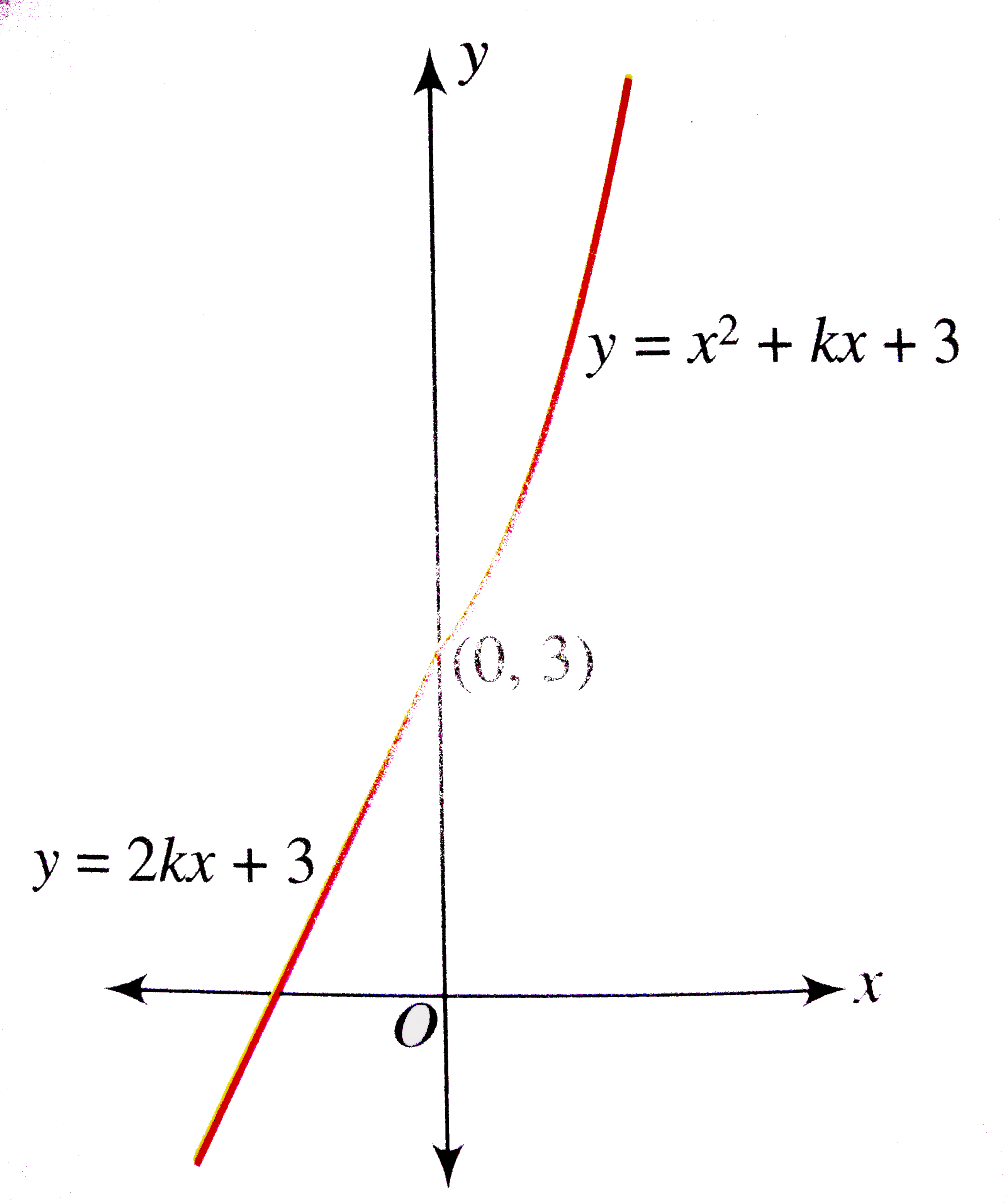
If F X Kx For X Le 2 3 For X Gt 2 Is Continuous At X 2 Then The Value Of K Is

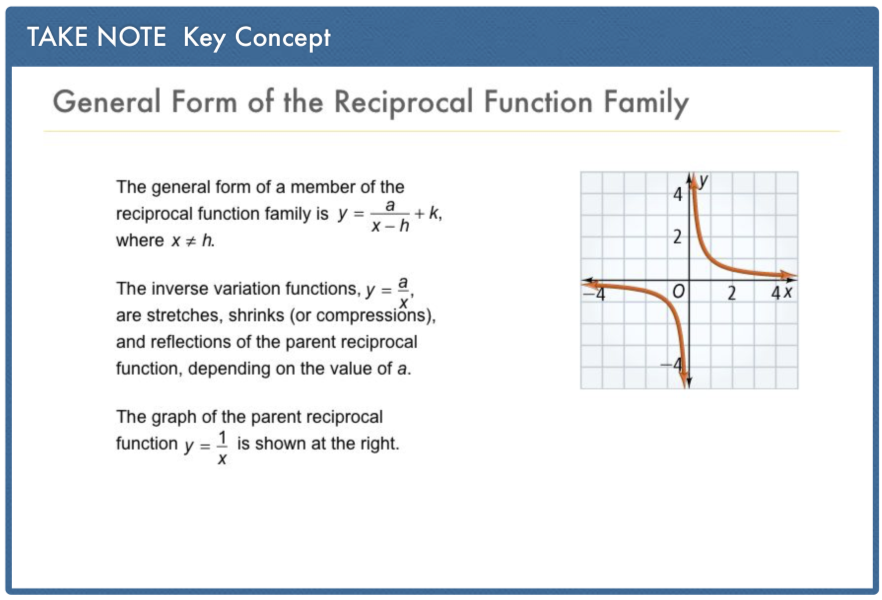
Algebra 2 8 2 Complete Lesson The Reciprocal Function Family Matthew Richardson Library Formative

Algebra Precalculus How Do You Explain That In Y X 2 Y Is Proportional To The Square Of X Mathematics Stack Exchange

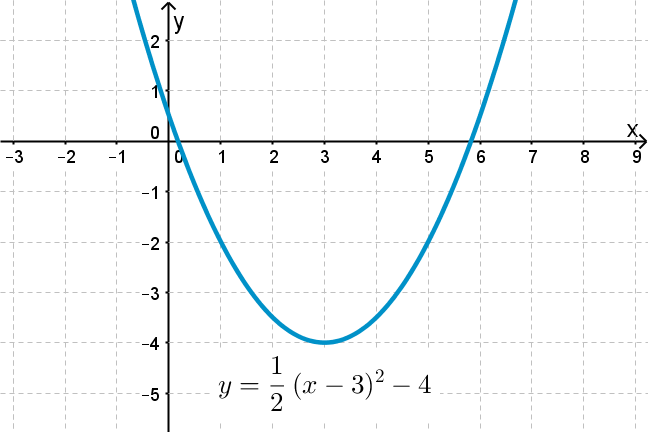
Scaling And Translating Quadratic Functions

Group Activity Graphing Direct And Inverse Proportions

3 4 Maximum Likelihood Estimator Mle Practical Econometrics And Data Science

2 5 The Graph Of Y Kx 2 Youtube

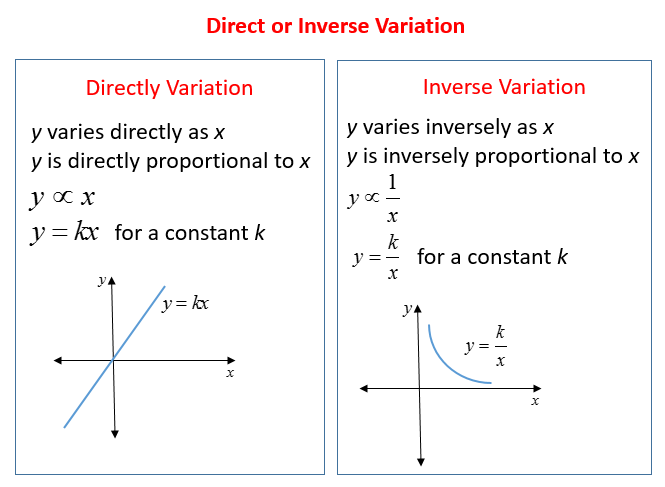
Direct Variation Video Lessons Examples And Solutions

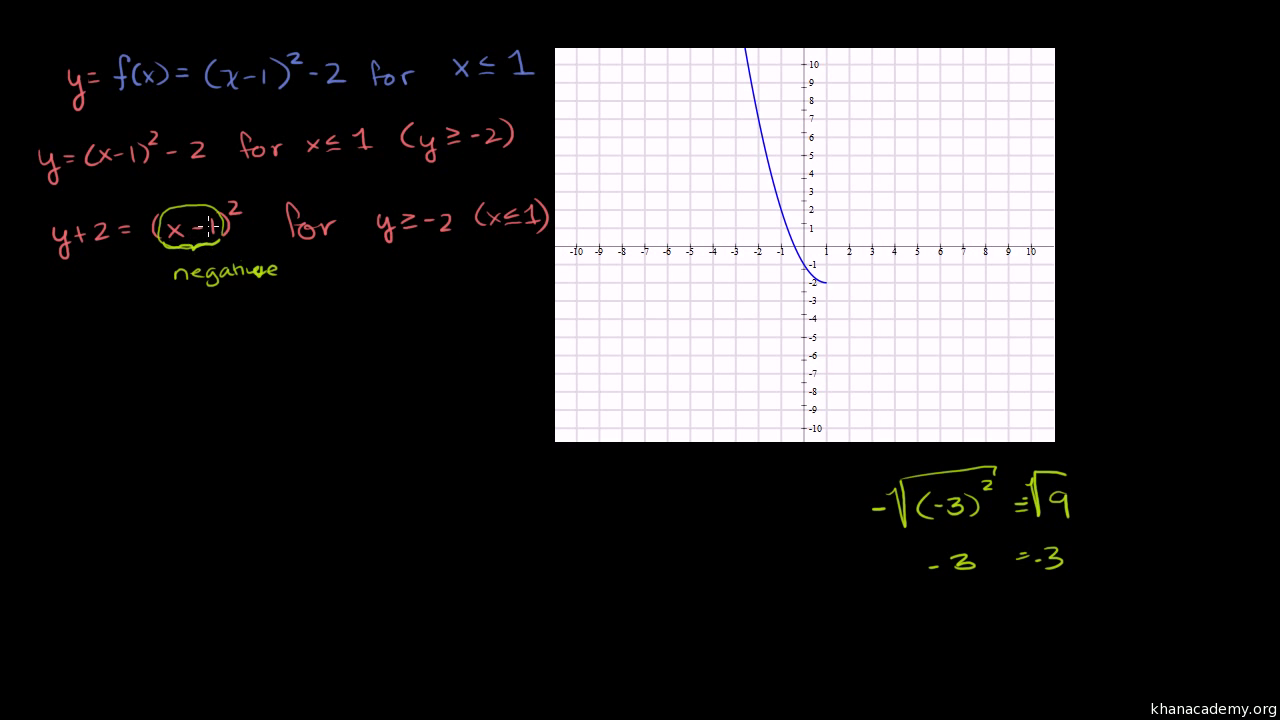
Finding Inverse Functions Quadratic Example 2 Video Khan Academy

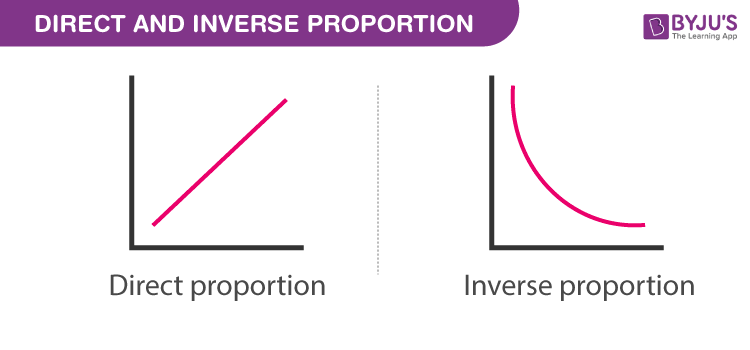
Inversely Proportional Definition Formula And Examples

Reciprocal Function Explanation Construction Of Graph And Solved Examples

All Elementary Mathematics Study Guide Functions And Graphs Elementary Functions And Their Graphs

2

Express Z Varies Directly With The Sum Of The Squares Of X And Y Solved

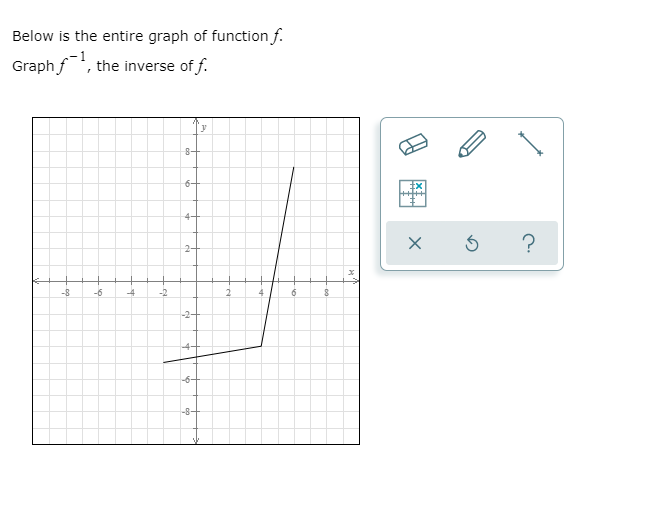
Below Is The Entire Graph Of Function F F The Chegg Com

Direct And Inversely Proportional Quantities Concepts Videos Examples

2

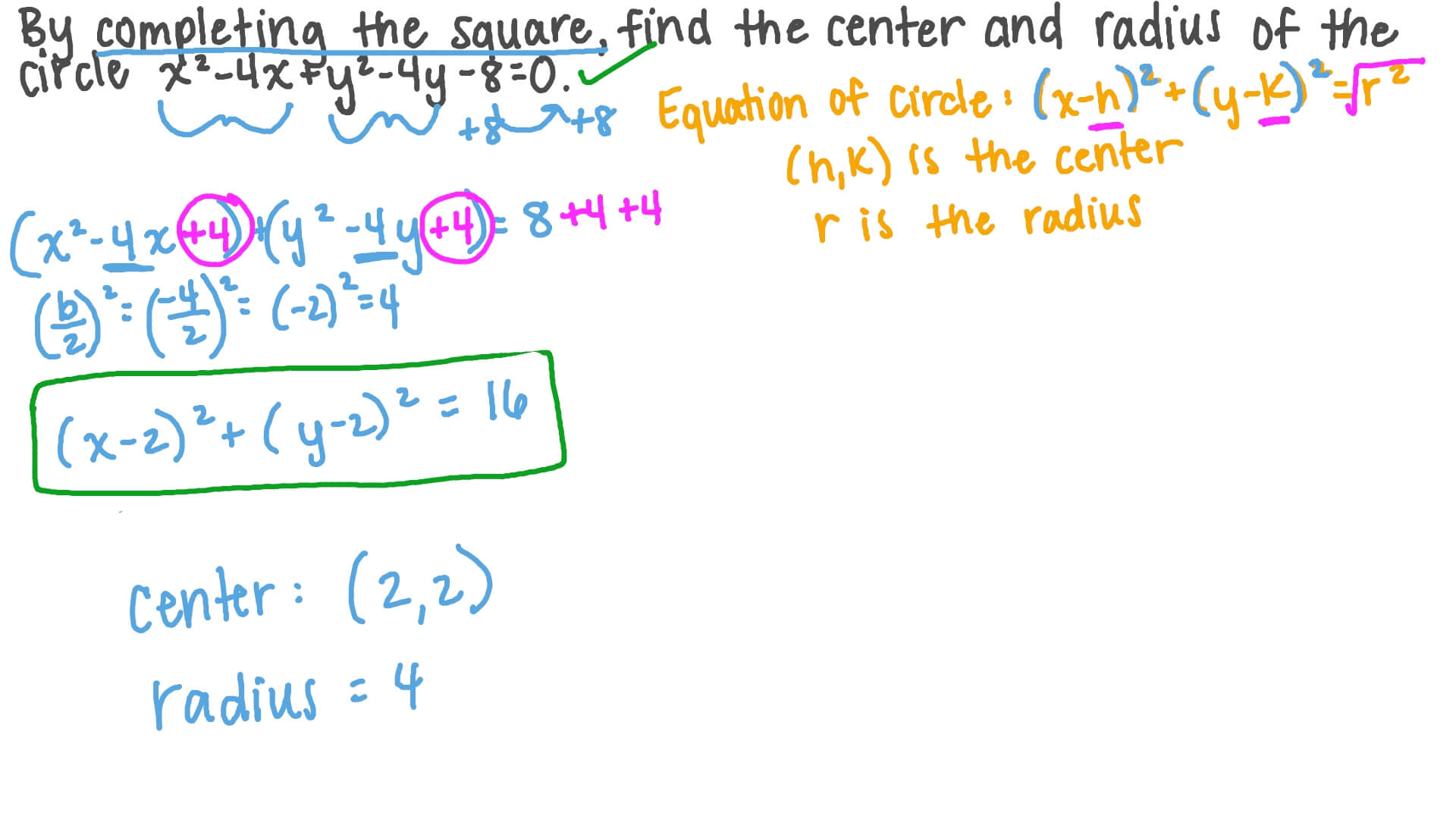
Question Video Finding The Center And Radius Of A Circle By Completing The Square Nagwa

Times Module M35 Proportion

2 02 Quadratic Equations

Direct Inverse Relationships Lab

Graph Quadratic Functions Using Properties Intermediate Algebra

What Does An Inverse Proportion Graph Look Like Quora

Integrated Rate Laws

Algebra Precalculus Basic High School Mathematics Graphing The Inverse Square Law Mathematics Stack Exchange

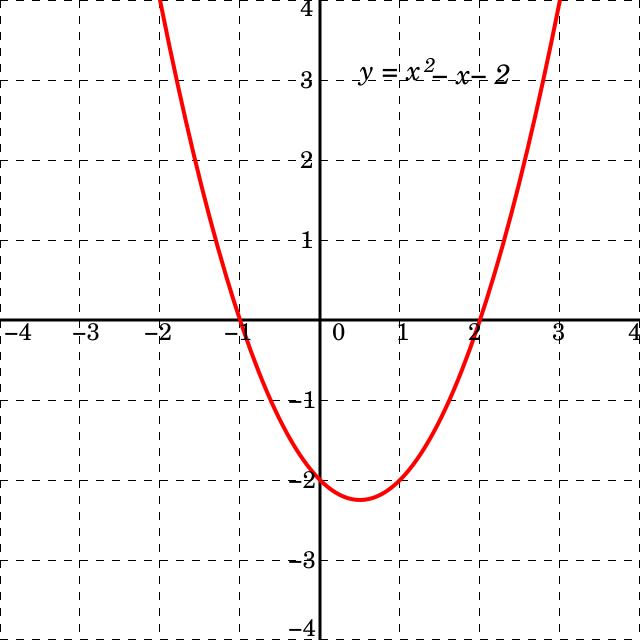
Quadratic Function Wikipedia

Directions Read Each Item Carefully Write The Le Gauthmath

2

2 02 Quadratic Equations

Variation College Algebra

Direct Variation Definition Examples Expii

Using Linear Quadratic Inverse Inverse Square Graphs To Understand Proportionality Ib Physics Youtube

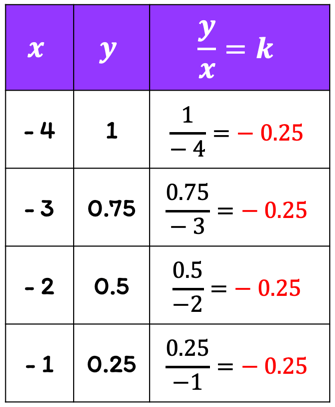
Direct Variation Chilimath

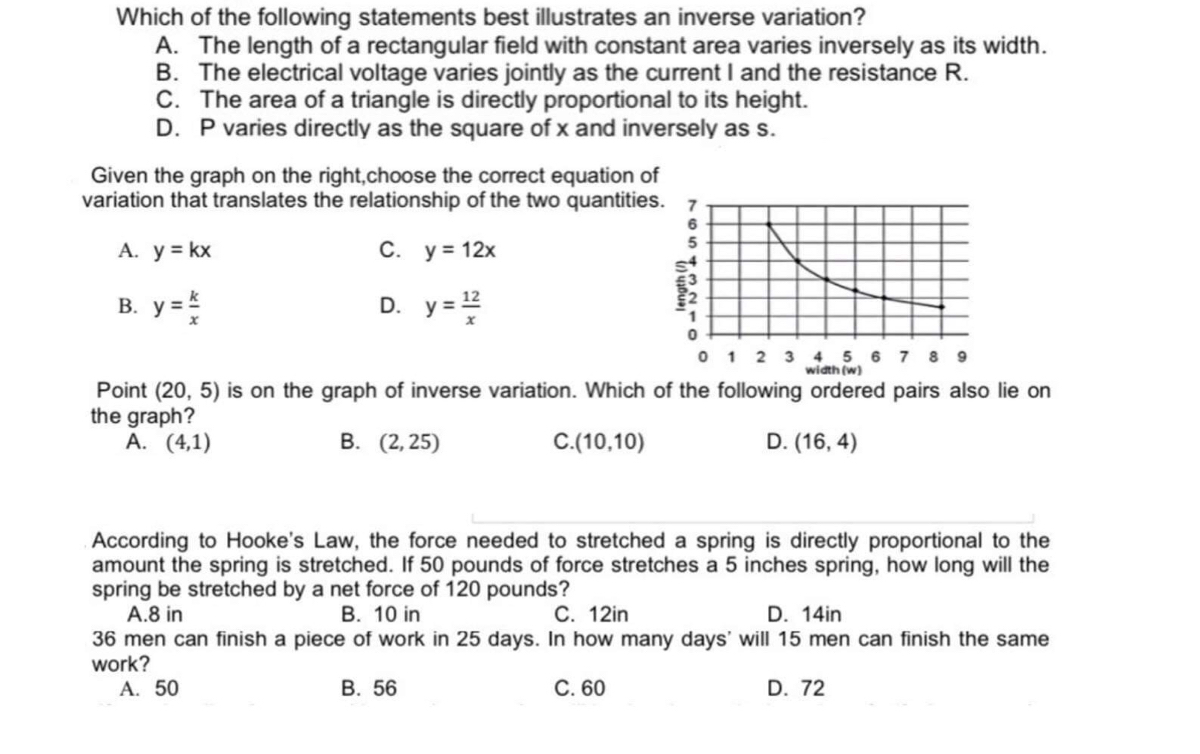
Answered Which Of The Following Statements Best Bartleby

Pplato Flap Phys 1 3 Graphs And Measurements

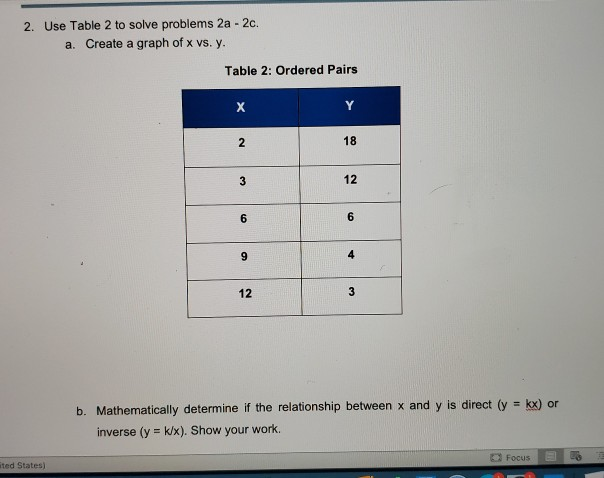
Solved 2 Use Table 2 To Solve Problems 2a 2c A Create A Chegg Com

What Is Meant By Direct Proportional And Inversely Proportional Quora

1

Inversely Proportional Definition Formula Examples Video Lesson Transcript Study Com

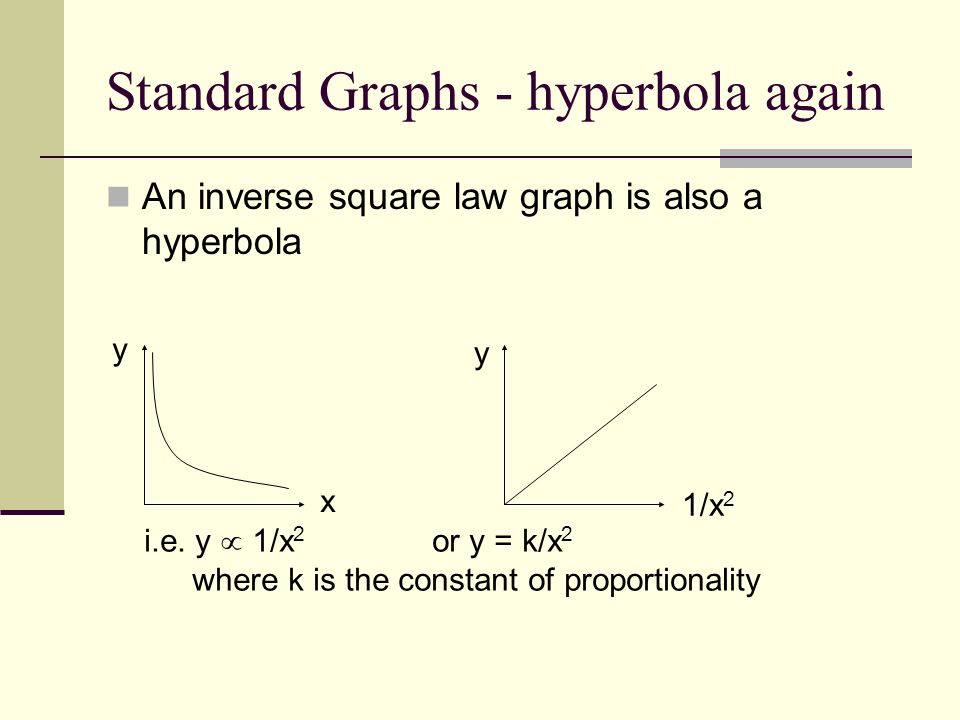
Physics And Physical Measurement Topic 1 2 Measurement And Uncertainties Ppt Download

Graph Graph Equations With Step By Step Math Problem Solver

Times Module M35 Proportion

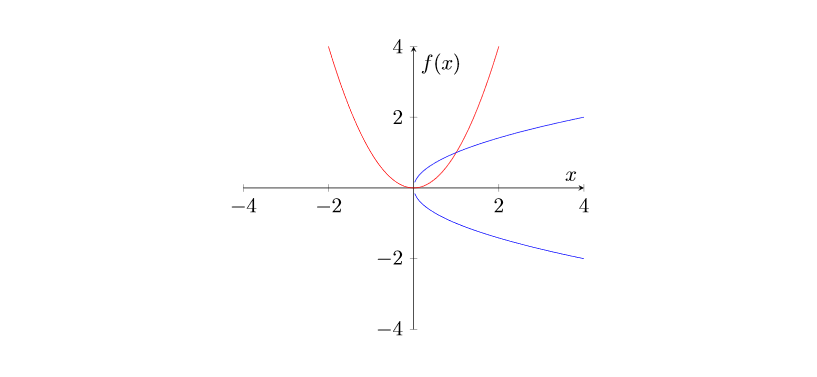
Inverse Quadratic Interpolation 1 Introduction By Samuel Harlen Medium

Mathematics Free Full Text Figures Of Graph Partitioning By Counting Sequence And Layer Matrices Html

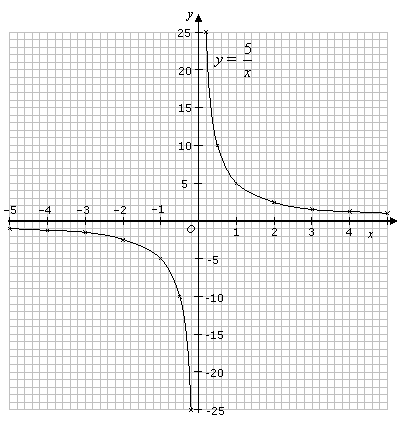
Graphs Of Reciprocal Functions Video Lessons Examples And Solutions

The Inverse Square Law 1 R And The Sound Intensity Sound Energy Quantity Not For Sound Pressure As Sound Field Quantity Acoustic Audio Sound Reduction Free Field Particle Amplitude Volume Loudness

1

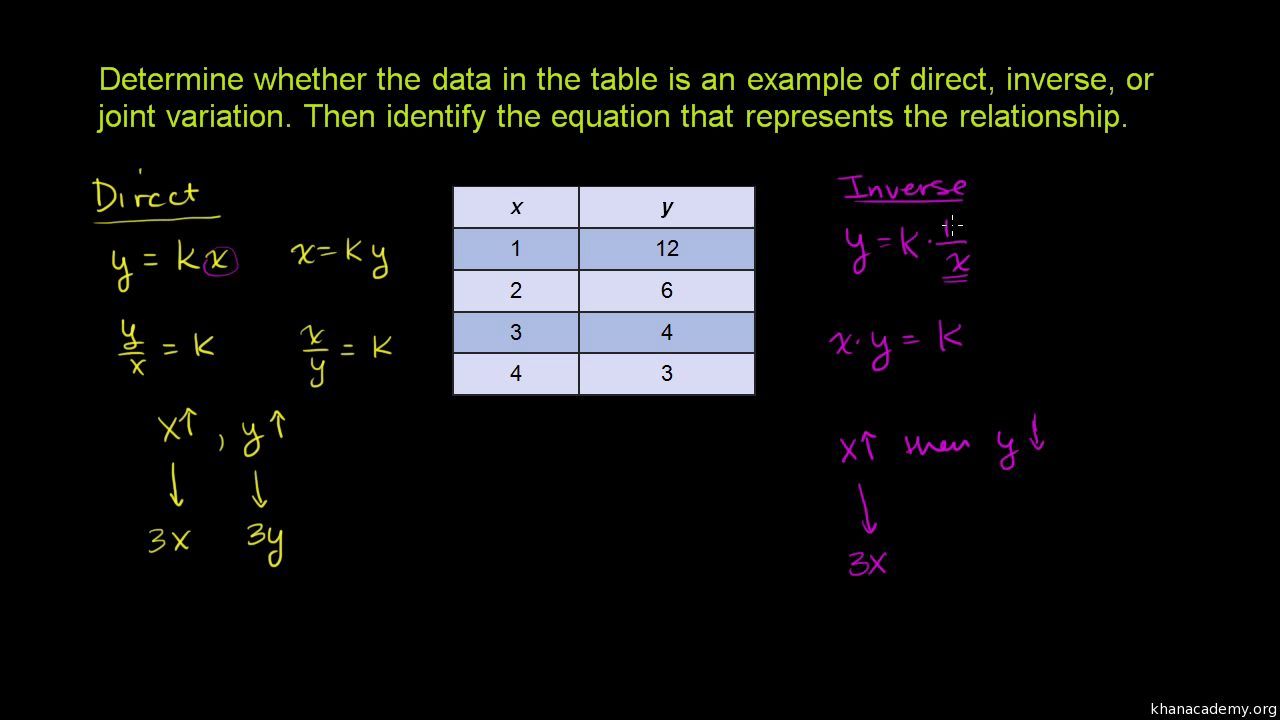
Recognizing Direct Inverse Variation Table Video Khan Academy

Inversely Proportional Definition Formula Examples Video Lesson Transcript Study Com

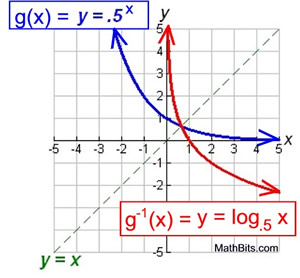
Exponential Functions Mathbitsnotebook Ccss Math

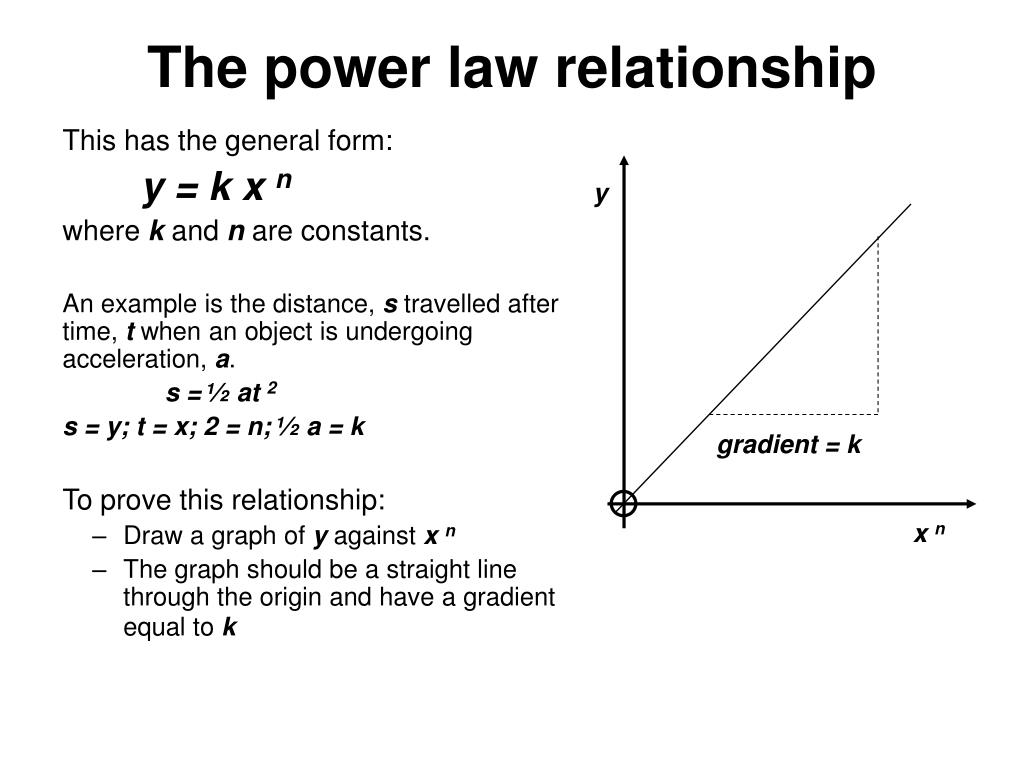
Ppt The Equation Of A Straight Line Powerpoint Presentation Free Download Id

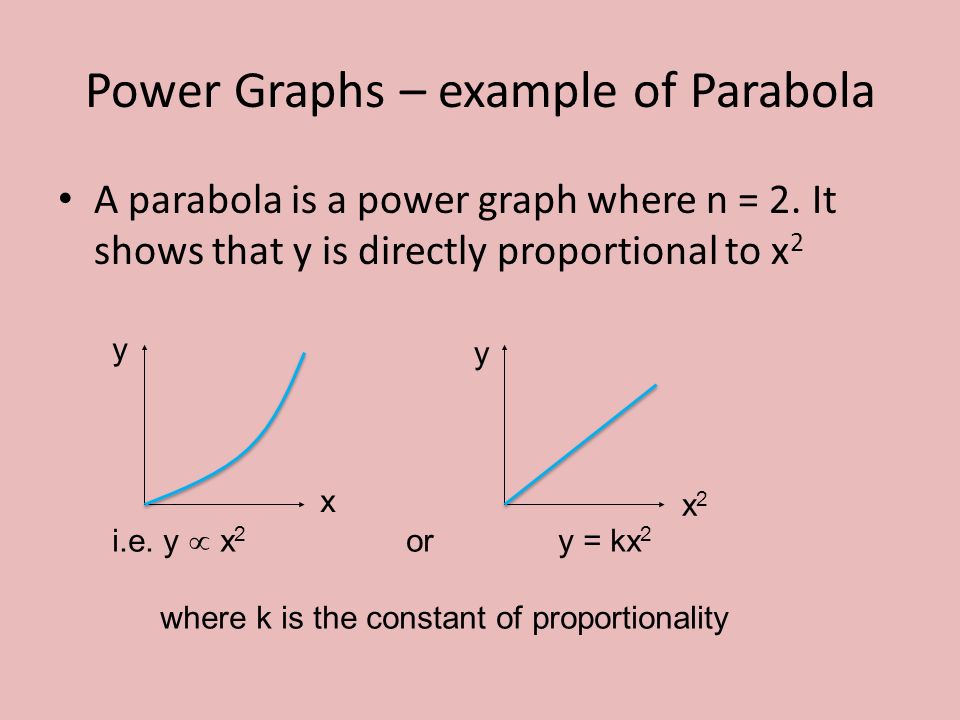
Proportional Techniques Standard Graphs Proportional Techniques Ppt Download

Chapter 3 1 Variation Ppt Video Online Download

Direct Inverse Joint And Combined Variation Math Hints

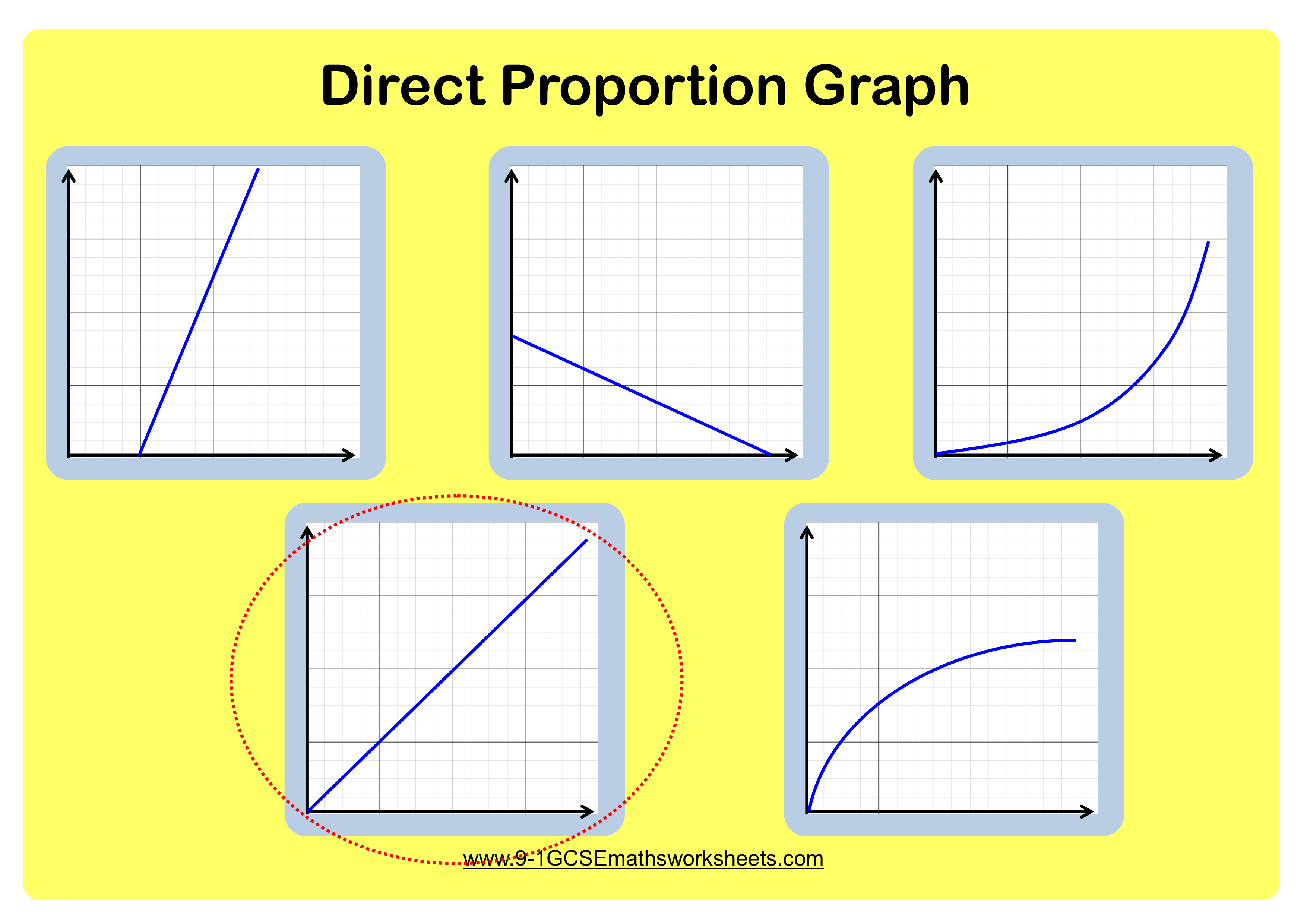
Direct Proportion Worksheets New Engaging Cazoomy

Rational Functions Algebra And Trigonometry

Ch 9 10

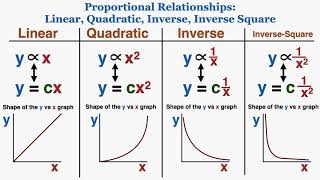
Using Linear Quadratic Inverse Inverse Square Graphs To Understand Proportionality Ib Physics Youtube

Instantcert Credit College Algebra Lesson 12

Incoming Term: inverse square y=k/x^2 graph,